Ion and Electron
Characteristics in Auroral Density Cavities Associated with Ion Beams:
No Evidence for Cold Ionospheric Plasma
Submitted to JGR, May 15, 1998
J. P. McFadden,
C. W. Carlson, and R. E. Ergun
Space
Sciences Laboratory, University of California, Berkeley, CA.
Lockheed
Martin Palo Alto Research Laboratory, Palo Alto, CA
University
of New Hampshire, Durham, NH
Low density
cavities associated with upgoing ion beams were identified in the auroral
regions over two decades ago. In order to understand the waves, double
layers, and solitary structures observed within these cavities, accurate
measurements of the plasma distribution function are required. Although
measurements by DE-1 indicated that these cavities were composed primarily
of hot plasma in the form of ion beams, plasma sheet ions, and inverted-V
electrons, later reports from Viking showed these cavities contained a
cold plasma component whose density was an order of magnitude larger than
the hot component. Recent measurements by the FAST satellite contrast sharply
with the Viking results and support the earlier DE-1 observations. Regions
of upgoing ion beams observed by FAST are shown to contain little or no
cold plasma. The hot electron densities (>100 eV) and the combined plasma
sheet ion and upgoing ion beam densities (>30 eV) agree remarkably well.
Furthermore, no cold (0-30 eV) ions are measured at low energies by the
mass spectrometer which precludes the presence of significant (>20%) cold
electrons to preserve charge neutrality. Characteristics of the plasma
for 11 ion beam events are tabulated.
The auroral upward
current regions contain low density cavities associated with upgoing ion
beams (Persoon et al., 1988; Strangeway et al., 1998). These cavities are
thought to be caused by the evacuation of plasma by field aligned potential
drops at lower altitudes (Mozer et al., 1977). Accurate measurement of
the particle distributions are required in order to characterize the waves,
double layers and solitary structures observed in these density cavities
(Kintner et al., 1978; Temerin et al., 1982; Koskinen et al., 1990). Accurate
particle measurements are also necessary to understand how the plasma is
able to support the parallel electric fields. This paper will identify
the primary particle distributions observed in these ion beam regions or
density cavities. Throughout this paper the term density cavity and ion
beam region can be considered synonymous, however we primarily use the
latter since historically these regions have been identified by the ion
sensors.
In an extensive
study using both wave and particle data from DE-1, Persoon et al. (1988)
concluded that the thermal (<18 eV) electrons and ions were minor components
of the plasma in density cavities associated with upgoing ion beams. Later
observations from the Viking spacecraft (Koskinen et al., 1990) indicated
that ion beam regions contained a cold (few eV) ion population that was
approximately an order of magnitude denser than the energetic ion beam.
Observations near their instrument sensitivity threshold also indicated
that the cold ions were drifting up the field line. No mass separation
was performed and the cold ions were assumed to be hydrogen. A cold (few
eV) electron component with roughly the same density was inferred from
Langmuir probe measurements (Bostrom et al., 1988; Koskinen et al., 1989)
that could provide charge neutrality. These Viking observations were made
at 8000-11000 km altitudes, in the afternoon-evening sector, and in aurora
with a total potential drop of about 1 kilovolt.
During the first
year of the FAST mission, numerous ion beams were identified at altitudes
>2500 km, typically in the nightside winter hemisphere. The FAST observations
described below were selected from a set of orbits near midnight, in the
northern winter hemisphere, at altitudes near 4000 km. Below we present
detailed observations from three ion beam regions and general properties
of the 11 events studied. Selection criteria for the events included ion
beams lasting >10 seconds, valid mass spectrometer data, and low amplitude
(<100 mV/m) electric fields. We will show that the hot ion and electron
densities agree within measurement uncertainties (~20%), and that no evidence
for cold plasma is observed. These results support the observations reported
by Persoon et al. (1988) and contrast sharply with Viking results.
The FAST satellite
contains top hat design, (Carlson et al., 1983; Carlson and McFadden.,
1998a) electrostatic analyzers to measure the ion (3 eV - 25 keV) and electron
(4 eV - 30 keV) distributions. The analyzers employ microchannel plate
detectors in chevron configuration and biased to produce narrow pulse height
distributions well above the preamplifier thresholds. The spacecraft is
in a reverse cartwheel orbit and oriented relative to the magnetic field
such that plasma analyzers measure full pitch angle distributions continuously.
Analyzers include deflectors at their entrance aperture that steer their
field of view by +/-10o to always include both the parallel
and anti-parallel magnetic field line. Both analyzers form a 48 energy
x 32 angle measurement each 78 ms. This study uses "survey data" formed
by onboard averaging software which provides continuous coverage across
the auroral regions. The electrostatic analyzers provide the bulk of the
measurements used in this study, so we include a discussion of instrument
calibration and measurement uncertainties below. A discussion of false
counts in ion sensors (background, UV contamination, scattered electrons)
is included in the "Observations" section where these sources of error
are obvious from the data.
Large electric
fields along the spacecraft velocity vector (approximately north-south)
can produce ExB drifts that move field aligned ion beams out of the analyzer's
360ox6o planar (or conical when deflectors are used)
field of view. This deflection introduces an error in the density calculation
of ion beams. The deflection is most pronounced for oxygen beams, where
a 100 mV/m field can deflect a 1 keV oxygen beam by about 5o for
magnetic fields at 4000 km. Although the deflection of hydrogen beams is
1/4 that of oxygen beams at the same energy, hydrogen beams tend to be
narrower (~10o FWHM) in pitch angle than oxygen beams (~30o
FWHM) so the effect on the pitch angle distribution measurement is similar.
A 5o deflection of a 1 keV proton beam requires a 400mV/m field
at 4000 km altitudes, and reduces the calculated ion density to about 0.7
of the actual density for a 10o FWHM beam.
East-west electric
fields also affect the ion density calculation by shifting the ion beam
off the magnetic field line. This error increases the calculated beam density
since a larger phase space volume is assumed in the density calculation.
Although east-west electric fields tend to be smaller, errors caused by
these fields can be larger. For the same 5o deflection of a
1 keV proton beam described above, the calculated ion beam density is about
1.6 times the actual density.
To reduce errors
in the ion density calculation, slowly varying ion beam events with small
electric fields (<100 mV/m during most of the ion beam) were selected
for the study. Errors introduced by these fields are believed to be the
major source of the small ~20% variations in the ratio of ion to electron
densities seen below. It may be possible to calculate the ion density by
correcting for large (>>100 mV/m) electric fields seen in the more turbulent
ion beams, however this type of modeling is beyond the scope of this paper.
The ion and electron
analyzer absolute geometric factors were estimated pre-flight from computer
simulation, known grid transparencies, and published values of microchannel
plate efficiency. The sensors were checked for uniformity using a Ni63
beta source and found to vary <20% around the analyzer field of view.
The electron sensor was also tested in-flight for uniformity and small
corrections in sensitivity with look direction are included in the calibration.
The absolute geometric factor was tested in-flight by comparing the measured
magnetic deflection across inverted-V arcs with the expected deflection
determined from the measured electron flux and found to agree nearly exactly.
(For examples see McFadden et al., 1998; Carlson et al., 1998b.)
The ion sensor
calibration was tested by comparing the hot (>100 eV) electron and hot
(>30 eV) ion densities in a selected ion beam (see Figure 1 and orbit 1616
in Table 1). A 35% adjustment to the pre-flight estimated sensitivity was
required to increase the calculated ion density to that of the measured
hot electron density. If cold electrons were present, a larger correction
factor would be required. We attribute this correction to uncertainties
in our knowledge of the absolute MCP efficiency for ions. Data from orbit
1616 were chosen because the plasma was primarily hydrogen (mass effects
were nearly negligible on the density calculation) and because low level
background counts due to scattered electrons in the ion sensor (see Observations
section) could be ignored. In addition, no cold (<30 eV) ions were observed
in the mass spectrometer, so the primary error in this normalization comes
from a lack of knowledge of the cold (<100 eV) electron density (see
Appendix: Cold Electron Measurements). The use of orbit 1616 data to normalize
the ion sensor geometric factor would appear to invalidate this orbit as
test for agreement between ion and electron densities. However, all 10
additional orbits showed good agreement between the hot ion and electron
densities and a similar correction to the ion spectrometer's geometric
factor would have been calculated for any of the 11 orbits. Since it is
unlikely that the ratio of cold to hot electrons was the same for all 11
ion beam events, we have confidence that our normalization method is sound.
Finally, an additional
source of error can arise from microchannel plate efficiency dependence
upon energy and mass (Burrous et al., 1967; Gao et al., 1984). The FAST
sensors include post-analyzer accelerations of ~450 V for electrons and
~1800 V for ions which should reduce these efficiency variations to the
<10% level.
The FAST satellite
also contains a Time-of-flight Energy-Angle imaging Mass Spectrometer (TEAMS)
which measures the 3-D ion distribution each spin (Moebius et al., 1998a).
These data, typically available with 5 second resolution, are used to obtain
the relative H+, He+, and O+ densities across the ion beams. Pre-flight
TEAMS calibrations are used for these data with estimated errors in relative
sensitivity versus mass of <20% and with absolute sensitivity knowledge
of ~50%. Since our calculations only use relative density versus mass to
correct the upgoing ion beam density for mass, and since these corrections
are typically a 25% density correction, TEAMS calibration errors should
introduce <5% errors.
The relative ion
densities vary slowly across arcs (Moebius et al., 1998b) and average ion
density ratios were used to correct the higher time resolution ion spectrometer
data for mass in the density calculation. The density ratio variations
were small for most of the selected events, and we estimate <10% error
introduced by changes in composition across these ion beams. However, two
of the ion beams (orbits 1761 and 1793, Table 1) showed a larger variation
in composition, which resulted in ~20% errors in the calculation during
portions of these beams. A more sophisticated composition correction would
have reduced this error, but was deemed unnecessary to improve the conclusions.
The mass spectrometer data are also used to show that low level background
counts in the ion spectrometer are due to internally scattered energetic
electrons.
Observations
Figure
1 shows a section of an inverted-V arc (orbit 1616) containing ion
beams that stretch over ~0.5o of invariant latitude. The top four panels
show electron energy, electron pitch angle, ion energy and ion pitch angle
spectrograms, respectively. The electrons have a spectral peak varying
slowly between 3 and 1 keV and are isotropic in pitch angle except for
the loss cone. Dropouts in the atmospheric secondary electrons (<100
eV, panel a) can be seen coincident with the appearance of the ion beam
(panels c and d). The ion beam varies slowly in energy between 30 eV and
1 keV, and is collimated to within about 10o of the magnetic
field. Panel e shows the hot (>100 eV) electron density calculated from
the measured distributions. The low energy (<100 eV) electron measurement
is dominated by spacecraft photoelectrons and scattered secondary electrons
precluding any reliable measurement of this density (see Appendix: Cold
Electron Measurements). Panels f and g show the hot ion beam (>30 eV, 150o-180o
pitch angle) and plasma sheet ion (>30 eV, 0o-150o pitch angle) densities
calculated from the measured distributions. As discussed below, the mass
spectrometer shows that no cold (<30 eV) ions are present in the ion
beam region. The density calculation requires a mass assumption (n ~ (count
rate) x  /
/  ) since the ion spectrometer is not mass resolving. The plasma sheet ion
density is calculated assuming hydrogen, which is consistent with the mass
spectrometer data. The ion beam density has been calculated using a mass,
) since the ion spectrometer is not mass resolving. The plasma sheet ion
density is calculated assuming hydrogen, which is consistent with the mass
spectrometer data. The ion beam density has been calculated using a mass, 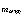 , given by:
, given by:
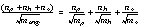
where np,
nh, no are the relative densities of hydrogen, helium,
and oxygen, determined by the mass spectrometer and averaged over the ion
beam. For this event, the above correction is  . Panel h shows the ratio of hot ion to hot electron densities which shows
remarkably good agreement between the densities inside the ion beam even
though the beam density (panel f) varies by a factor of ~5 and the electron
density (panel f) varies by a factor of ~2.
. Panel h shows the ratio of hot ion to hot electron densities which shows
remarkably good agreement between the densities inside the ion beam even
though the beam density (panel f) varies by a factor of ~5 and the electron
density (panel f) varies by a factor of ~2.
Now consider the
measured ion distributions and why we introduced a cutoff of 30 eV in the
ion density calculation.
Figure 2a shows
a contour plot of the ion differential energy flux (proportional to sensor
count rate) for a 10 second average during the ion beam in Figure
1. The beam at 180o pitch angle, and the hot, nearly isotropic,
plasma sheet ions are easily identified. The average counts per energy-angle
bin below 100 eV is about 1 in the contour plot. The slight enhancement
in low energy counts at pitch angles between 180o and 270o
is due to scattered ultraviolet into the sensor. Figure 2b and 2c show
the differential flux and count rate for this event, summed over pitch
angle. Note that the count rate (2c) is roughly constant, or independent
of energy, below 50 eV and forms a differential flux spectra (2b) proportional
to 1/energy. Figure 2d shows the partial ion density at each energy step
assuming H+ (blue line,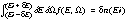 , where Ei
is the center energy of each energy step and
, where Ei
is the center energy of each energy step and 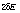 is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line, 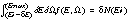 , where
Emax is the highest energy step). One can see that most of the density
resides in the hot component, however as much as 20% appears to exist in
the cold ions (4-30 eV). These low energy counts are about an order of
magnitude above the microchannel plate noise levels. However, as shown
below, these counts are due to a small number of scattered energetic electrons
and a small amount of solar ultraviolet and do not represent actual cold
plasma.
, where
Emax is the highest energy step). One can see that most of the density
resides in the hot component, however as much as 20% appears to exist in
the cold ions (4-30 eV). These low energy counts are about an order of
magnitude above the microchannel plate noise levels. However, as shown
below, these counts are due to a small number of scattered energetic electrons
and a small amount of solar ultraviolet and do not represent actual cold
plasma.
In addition to
the ion spectrometer, the FAST satellite has a mass spectrometer which
covers roughly the same energy range. The overall geometric factor of the
mass spectrometer is about one half that of the ion spectrometer, producing
count rates that differ by a factor of ~2 between the instruments. Figure
3a shows the count rate, summed over angle, from the mass spectrometer
for the same interval as Figure 2. Total
counts from the ion beam are smaller by a factor of ~50 for the mass spectrometer
since it samples the ion beam only twice per spin whereas the ion spectrometer
samples the beam continuously. A better comparison uses the nearly isotropic
plasma sheet ion count rates (>5 keV) which differ by ~2 as expected for
the two instruments.
In contrast to the
ion spectrometer, the mass spectrometer measures no counts below about
40 eV. This is because the mass spectrometer is not susceptible to scattered
electrons (or sunlight) due to coincidence detection in the time-of-flight
electronics and due to a large (-20 kV) post acceleration between the analyzer
and time-of-flight detector (Moebius et al., 1998a). Examination of ion
beam events has shown that the ion spectrometer's low energy count rate
increases with electron flux and electron energy, whereas the mass spectrometer
observes no counts at these energies. For the event in Figure
1, false counts due to scattered electrons are rather small because
most of the inverted-V electrons are repelled by the -1800 V bias on the
front of the ion spectrometer's microchannel plate. In energetic arcs (~10
keV), the scattered electron count rate can be an order of magnitude larger.
For these reasons, density calculations using the ion spectrometer do not
include the low energy (<30 eV) counts due to scattered electrons, which
is consistent with no counts in the mass spectrometer data at these energies.
Figure
3b uses the same format as Figure 2d
to display the partial ion density at each energy step assuming H+ (blue
line), and the partial integral of the density starting at the highest
step (green line) for the TEAMS measurement. Here we have summed the counts
at all masses and assumed H+ to mimic the ion spectrometer measurement,
but have used the full 3-D TEAMS measurement. A comparison of the two density
calculations show quite good agreement (only 20% difference when the <30
eV counts are ignored) between instruments with different sensitivities,
field-of-views, and detection systems.
Before proceeding
with additional examples, a final word should be said about the possible
presence of cold ions whose energy is below the mass spectrometer's lower
energy threshold of 2-5 eV (which depends upon spacecraft potential), and
the possibility of cold electrons below our 100 eV cutoff. First, it would
be quite remarkable if this plasma existed, since it would require both
the cold electron and cold ion populations to be present with the same
density, independent of hot plasma densities. However, the proof comes
from the presence of large (~1 V/m) perpendicular electric fields in the
stronger ion beams not used in this study. The ExB drifts from these fields
would cause cold hydrogen (oxygen) to have energies of tens (hundreds)
of eV in the spacecraft frame. Low energy cold ions moving perpendicular
to B would be easily detected by FAST and have not been observed during
these strong electric fields. The lack of cold ions precludes the presence
of significant cold electrons to preserve charge neutrality. In addition,
Strangeway et al. (1998) has shown that the hot electron density is consistent
with measured plasma wave cutoffs, and Ergun et al. (1998) has shown that
the AKR spectrum is consistent with a hot, relativistic electron population,
providing further proof that the cold plasma is a small fraction of the
total density.
Figure
4 shows an example of an ion beam event from orbit 1676 with the same
format as Figure 1. The top 2 panels show
an isotropic inverted-V electron distribution whose energy is just above
1 keV. The ion beam (panels c and d) varies between 100 eV and 2 keV. Low
energy ion conics are also observed at 10:05:41-45 and after 10:05:49.
Panel e shows the hot (>100 eV) electron density and panels f and g show
the ion beam (>30 eV, 150o-180o pitch angle) and plasma sheet ion (>30
eV, 0o-150o pitch angle) densities. The ion beam
density calculation used 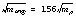 as determined
from the mass spectrometer data. The jump in plasma sheet ion density (panel
g) at 10:05:41-:45 and after 10:05:49 is caused by ion conics included
in the integral. Panel h shows that the ratio of hot ion to hot electron
density is about 1 when the ion beam is present.
as determined
from the mass spectrometer data. The jump in plasma sheet ion density (panel
g) at 10:05:41-:45 and after 10:05:49 is caused by ion conics included
in the integral. Panel h shows that the ratio of hot ion to hot electron
density is about 1 when the ion beam is present.
The smaller flux
of inverted-V electrons in Figure 4a, as
compared to
Figure 1a, resulted in a factor
of 3 reduction in scattered electrons into the ion sensor. Figure
5 shows a 10 second average of the ions in a format identical to Figure
2. Figure 5c shows a relatively constant count rate below 50 eV, similar
to that in Figure 2c but with a lower rate. Mass spectrometer data for
this beam shows no ions below 40 eV indicating these counts are instrumental.
As can be seen from Figure 5d, the counts
resulting from scattered electrons would have resulted in only a ~20% error
for the ion density.
Figure
6 shows a third example of an ion beam event, this time from orbit
1771. The format is the same as Figures 1 and 4. The top 4 panels show
an isotropic inverted-V electron population with an energy of ~1 keV, and
an ion beam varying from about 100 eV to 1 keV. The mass spectrometer gave
a beam mass of 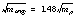 for the beam density calculation
(panel f). The plasma sheet ion density (panel g) is almost negligible
(~0.05 cm-3) for this event. Although the ion beam density (panel
f) varies by nearly a factor of 8, panel h shows that the ratio of hot
ion to hot electron densities remains near one across the event.
for the beam density calculation
(panel f). The plasma sheet ion density (panel g) is almost negligible
(~0.05 cm-3) for this event. Although the ion beam density (panel
f) varies by nearly a factor of 8, panel h shows that the ratio of hot
ion to hot electron densities remains near one across the event.
A comparison of
ion beams on the three orbits (Figures 1, 4, and 6) shows a number of differences
and similarities between the ion beams. The density was highest for orbit
1616, varying from about 1 to 3 cm-3, whereas the beam density
on orbit 1676 remained below 0.6 cm-3. Orbit 1771 showed the
largest variations in density swing from 0.2 to 1.1 cm-3. Slightly more
oxygen was present during orbits 1676 and 1771, as seen from the beam mass
correction or Table 1. The additional oxygen also shows up in contour plot
5a as the wider pitch angle portion of the beam at higher energies. In
all three events the plasma sheet ions have a nearly constant density across
the event, whereas the ion beam shows large density variations. To first
order the beam density variations reflect conservation of flux, where a
relatively constant flux of upgoing ions into the acceleration region should
show a density inversely proportional to the  .
.
Table
1 summarizes characteristics of the electrons and ions for 11 ion beam
events investigated in detail. All these events give good agreement between
the hot ion and hot electron densities. Orbit 1623 was the lowest energy
event and the lower limit for the ion beam calculation was dropped to 15
eV for this event. Most of the ion beam events had densities less than
1 cm-3, and the lowest observed density was 0.13 cm-3.
Variations in the O+/H+ and He+/H+ beam density ratios were significant
(greater than a factor of 2) for many of the events, especially orbit 1761
where a factor of 5 variation in O+/H+ was observed across the ion beam.
However, the mass correction to the beam density for this event, 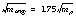 , varied by only +21% to -22% across the event due to additional changes
in He+/H+, and was relatively constant across most of the event giving
good agreement between ion and electron densities. A more sophisticated
program that uses a time varying,
, varied by only +21% to -22% across the event due to additional changes
in He+/H+, and was relatively constant across most of the event giving
good agreement between ion and electron densities. A more sophisticated
program that uses a time varying, 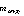 , across
the beams could have produced limited improvement in a couple of the density
ratio calculations but was unnecessary for this study. Table
1 provides theorists a range of parameters that can be used to model
the ion beam regions.
, across
the beams could have produced limited improvement in a couple of the density
ratio calculations but was unnecessary for this study. Table
1 provides theorists a range of parameters that can be used to model
the ion beam regions.
Discussion
The results presented
above show convincing evidence that the hot electron and hot ion densities
in auroral ion beam regions provide the bulk of the total density. The
measurements were made by high resolution electron and ion spectrometers,
with mass corrections provided by a time-of-flight mass spectrometer. The
primary errors in this measurement appear to be introduced by large, low
frequency electric fields, or electrostatic shocks, that deflect the ion
beam transverse to the field line due to ExB drifts. These deflections
result in <20% variations in the calculated density ratio on short time
scales, however the density ratio averaged over the events agree to better
than 10%. The cold ion (<30 eV) measurement is difficult with a spectrometer
because a small fraction of energetic electrons can scatter through the
analyzer producing false counts that corrupt the low energy measurement.
This contamination is not present in the mass spectrometer data, and these
data were used to show that no measurable ion fluxes were present below
30 eV. The detection of small fluxes of cold (<100 eV) electrons is
very difficult to make with a spectrometer due to spacecraft photoelectrons
and secondary electrons produced in the analyzer aperture (see Appendix:
Cold Electron Measurements), so only the hot electron density was calculated.
However, to preserve charge neutrality, we infer that no significant cold
electron population is present during ion beams. This result is consistent
with ion beam regions being produced by quasi-static parallel electric
fields, which prevent cold electrons from penetrating into the density
cavities associated with ion beams. In addition, wave observations by Strangeway
et al. (1998) and Ergun et al. (1998) have shown that plasma wave cutoffs
are also consistent with a hot (>100 eV) electron population providing
the bulk of the total density.
The evidence for
a lack of cold plasma in the density cavity supports the DE-1 results presented
by Persoon et al. (1988), but differs from previous reports of Viking data.
Koskinen et al. (1990) report evidence of a low energy, cold ion component
moving up the field line during ion beam events. The density of the cold
ion component was estimated to be an order of magnitude larger than the
hot ion beam. Figure 6 of the Koskinen et al. (1990) paper shows ion spectra
that resulted in the identification of cold plasma. The spectra below 100
eV varies as 1/energy, which implies a constant count rate similar to Figures
2c and 5c of this paper. Koskinen et al. (1990) conclude that the measurement
is valid since the low energy counts are significantly above their instrument
sensitivity. However, no discussion of possible sources of false counts
is included in the paper, specifically the instrument's sensitivity to
scattered energetic electrons. The Viking measurement of the low energy
ion flux is an order of magnitude larger than the false count rate in the
FAST ion spectrometer, and 2 orders of magnitude above the sensitivity
of the FAST mass spectrometer which saw no flux at these energies.
To provide charge
neutrality, Koskinen et al. (1990) report that a cold electron component
was also present during the ion beams with about the same density as the
cold ion component. This result was based upon Langmuir probe sweeps near
the ion beams which inferred a 1-10 eV temperature for the electrons (Bostrom
et al., 1988). However, Hilgers et al. (1992) shows that the Viking Langmuir
probe current is dominated by photoelectrons from the wire boom within
ion beam regions. They conclude that only an upper limit to the thermal
electron density can be determined and that cold electrons are at most
of the order of the energetic electron density.
It may be possible
that differences between the FAST and Viking observations are due to other
effects. Both data sets were measured at similar local times, with Viking
observations in the afternoon-evening sector and FAST observations at ~22
MLT. Viking measurements were made at higher altitudes (8000-11000 km),
typically above most of the acceleration region, whereas FAST observations
(~4000 km) are near the bottom of the acceleration region. However, there
are no reported observations or theories that suggest the plasma density
should increase with altitude inside the ion beam regions, with the lowest
densities at the bottom of the density cavities. The Viking observations
were made near solar minimum, as were the FAST observations. Koskinen et
al. (1990) state that the events were from "relatively weak acceleration
events associated with low geomagnetic activity". As seen from Table 1,
most of the FAST events were also during low activity with relatively weak
acceleration (~1-2 keV ion beams). In both cases the O+ beams tended to
have a higher energy and broader pitch angle width than the H+ beams. Koskinen
et al. (1990) report a relatively small (<30%) heavy ion density in
the upflowing ion beams. As seen from Table 1, the FAST observations differ
in that the sum of the He+ and O+ densities typically exceeded the H+ density
for the 11 events. These are typical of ion beams reported by Moebius et
al. (1998b) in a statistical study of FAST ion beam events. The Viking
events were also selected for a study of solitary waves. The 11 FAST events
were examined and solitary wave structures were found in all the beams.
From the above analysis and comparison, which suggests measurements of
similar phenomena under similar conditions, we conclude that the interpretation
of Viking measurements as observations of cold plasma in ion beam regions
are in error.
The new FAST observations,
which find only hot plasma in the ion beam regions, have strong implications
for the density profile and plasma waves in the upward current regions.
Without cold plasma, certain wave modes will disappear and other modes
grow without heavy damping by the cold electrons. Studies of wave modes
and solitary structures within ion beam regions that assumed a cold background
plasma (Marchenko and Hudson, 1995, and references therein) need to be
revisited in light of these new measurements. The need to postulate anomalous
resistivity to prevent current runaway may also be unnecessary since the
parallel acceleration region appears to contain only hot plasma. The measurements
are consistent with simple acceleration by parallel electric fields which
prevent cold ionospheric and secondary electrons from penetrating into
the acceleration region, and accelerate all the cold ions (or cool ion
conics) into energetic ion beams. The density profile along the magnetic
field line appears to be a simple function of the parallel electric field
profile, the high altitude plasma distribution, and the ionospheric plasma
distributions. It may be possible to determine the electric field profile
directly from the low and high altitude plasma distributions, combined
with charge neutrality and current continuity in a region carrying an upward
current. It is hoped that these new measurements will allow a consistent
theory to be developed to explain the parallel electric fields and waves
in the upward current regions.
Appendix: Cold Electron Measurements
Low energy electron
measurements in the auroral zone are extremely difficult due to spacecraft
photoelectron and secondary electron contamination. This appendix is included
to demonstrate the difficultly in this measurement, to identify the dominant
sources of low energy electron counts, and to justify the 100 eV cutoff
that was used.
Figure 7 shows contour plots
of the electron differential energy flux (proportional to count rate) both
inside (7a) and outside (7b) the ion beam of Figure 1. There are 6 primary
sources of counts below 100 eV: background microchannel plate noise, solar
ultraviolet scattering into the sensor, spacecraft generated photoelectrons,
internally scattered energetic electrons from the auroral electron beam,
trapped plasma electrons outside the loss/antiloss cone, and atmospheric
secondary electrons in the loss/antiloss cone. Only the last two sources
of counts represent plasma that should be used in the density calculation.
Microchannel plate background noise caused by radioactive decay and penetrating
radiation are negligible. Orientation of the analyzer and blackened, scalloped
analyzer hemispheres reduce solar ultraviolet to negligible levels also.
Spacecraft photoelectrons can be seen in both distributions near 90o
and
270o pitch angles. These electrons disappear when the spacecraft
is in shadow, but dominate the low energy measurement in sunlight. Scattered
electrons from the primary auroral electron beam dominate the count rate
during ion beams (7a) at pitch angles near 0o and 180o.
Atmospheric secondary and trapped electrons dominate the count rate when
the spacecraft is below the ion beam regions (7b), except at the lowest
energies and near 90o pitch angles where spacecraft photoelectrons
always dominate.
From the above
example, one can see that a quantitative low energy electron measurement
is difficult at best. Since low energy counts can dominate the density
calculation due to weighting by 1/ in the density
calculation, a spectrometer determined density measurement that includes
low energy electrons requires close scrutiny. Figure 7c, formed from the
distribution in 7a within the ion beam, shows the partial electron density
at each energy step (blue line,
in the density
calculation, a spectrometer determined density measurement that includes
low energy electrons requires close scrutiny. Figure 7c, formed from the
distribution in 7a within the ion beam, shows the partial electron density
at each energy step (blue line,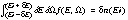 , where Ei
is the center energy of each energy step and
, where Ei
is the center energy of each energy step and  is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line, 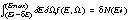 , where
Emax is the highest energy step). The contribution of photoelectrons and
internally scattered secondary electrons to the density clearly becomes
significant below 50 eV. The plateau of the green curve between 50 and
300 eV suggests minimal contribution of real electrons to the total density
at these low energies. This can be contrasted with Figure 7d, formed from
the distribution in 7b, which shows that atmospheric secondary electrons
between 50 and 300 eV contribute significantly to the total local density
outside ion beams.
, where
Emax is the highest energy step). The contribution of photoelectrons and
internally scattered secondary electrons to the density clearly becomes
significant below 50 eV. The plateau of the green curve between 50 and
300 eV suggests minimal contribution of real electrons to the total density
at these low energies. This can be contrasted with Figure 7d, formed from
the distribution in 7b, which shows that atmospheric secondary electrons
between 50 and 300 eV contribute significantly to the total local density
outside ion beams.
Although we neglect
the low energy (<100 eV) electrons in the density calculation due to
instrumental reasons, this assumption can be justified if the ion beams
are formed by quasi-static parallel electric fields. In this case cold
plasma should be excluded from the ion beam regions. One can also estimate
the density of neglected atmospheric secondary and trapped electrons with
energy <100 eV within an ion beam by folding an electron distribution
measured outside the ion beam through a 1 kilovolt potential. The potential
eliminates portions of the initial distribution below 1 keV, and adiabatically
maps the measured distribution between 1 and 1.1 keV to the 0 to 100 eV
energies. The calculated <100 eV density for this mapped distribution
is only a few percent of the hot (>100 eV) electron density. Since the
observed hot electron density agrees with the ion density as shown in Figures
1, 4, and 6, our assumption of negligible cold (<100 eV) plasma appears
to be justified. We note that the assumption that the <100 eV electrons
can be neglected is not valid when the ion beam has a low energy since
significant secondary electrons can then penetrate the potential below
the spacecraft. This effect can be seen in Figure 1 at 20:53:34, where
the ion beam density (panel f) goes up, and is not balanced by the hot
electrons (panel e).
The
analysis of FAST data was supported by NASA grant NAG5-3596.
Bostrom, R., G.
Gustafsson, B. Holback, G. Holmgren, H. Koskinen, and P. Kintner, Characteristics
of solitary waves and weak double layers in the magnetospheric plasma,
Phys. Rev. Lett., 61, 82, 1988.
Burrous, C. N., A. J. Lieber,
and V. T. Zavansteff, Detection efficiency of a continuous channel electron
multiplier for positive ions, Rev. Sci. Instrum., 38, 1477, 1967.
Carlson, C. W., D. W. Curtis,
G. Paschmann, and W. Michael, An instrument for rapidly measuring plasma
distribution functions with high resolution, Adv. Space Res., 2, 67, 1983.
Carlson, C. W., and J. P. McFadden,
Design and applications of imaging plasma instruments, AGU Monog. Meas.
Techn. Space Plasmas, ed. R. Pfaff, in press, 1998a.
Carlson, C. W., J. P. McFadden,
R. E. Ergun, M. Temerin, W. Peria, F. S. Mozer, D. M. Klumpar, E. G. Shelley,
W. K. Peterson, E. Moebius, R. Elphic, R. Strangeway, C. Cattell, R. Pfaff,
FAST observations in the downward auroral current region: energetic upgoing
electron beams, parallel potential drops, and ion heating, Geophys. Res.
Lett., in press, 1998b.
Ergun, R. E., C. W. Carlson,
J. P. McFadden, F. S. Mozer, G. T. Delory, W. Peria, C. C. Chaston, M.
Temerin, R. Elphic, R. Strangeway, R. Pfaff, C. A. Cattell, D. Klumpar,
E. Shelley, W. Peterson, E. Moebius, L. Kistler, FAST satellite wave observations
in the AKR source region, Geophys. Res. Lett., in press, 1998.
Gao, R. S., P.
S. Gibner, J. H. Newman, K. A. Smith, and R. F. Stebbings, Absolute and
angular efficiencies of a microchannel-plate position-sensitive detector,
Rev. Sci. Instrum., 55, 1756, 1984.
Gurnett, D. A. and
L. A. Frank, VLF hiss and related plasma observations in the polar magnetosphere,
J. Geophys. Res., 77, 172, 1972.
Hilgers, A., B.
Holback, G. Holmgren, and R. Bostrom, Probe measurements of low plasma
densities with applications to the auroral acceleration region and auroral
kilometric radiation sources, J. Geophys. Res., 97, 8631, 1992.
Kintner, P. M., M.
C. Kelley, and F. S. Mozer, Electrostatic hydrogen cyclotron waves near
one earth radius altitude in the polar magnetosphere, Geophys. Res. Lett.,
5, 139, 1978.
Koskinen, H., R.
Bostrom, and B. Holback, Viking observations of solitary waves and weak
double layers on auroral field lines, in Ionosphere-Magnetosphere-Solar
Wind Coupling Processes, edited by T. Chang, G. B. Crew, and J. R. Jasperse,
p.147, Scientific, Cambridge, Mass., 1989.
Koskinen, H., R.
Lundin, B. Holback, On the plasma environment of solitary waves and weak
double layers, J. Geophys. Res., 95, 5921, 1990.
Marchenko, V. A.,
M. K. Hudson, Beam driven acoustic solitary waves in the auroral acceleration
region, J. Geophys. Res., 100, 19791, 1995.
McFadden, J. P.,
C. W. Carlson, R. E. Ergun, C. C. Chaston, F. S. Mozer, M. Temerin, D.
M. Klumpar, E. G. Shelley, W. K. Peterson, E. Moebius, L. Kistler, R. Elphic,
R. Strangeway, C. Cattell, R. Pfaff., Electron modulation and ion cyclotron
waves observed by FAST, Geophys. Res. Lett., in press, 1998.
Moebius, E., L.
M. Kistler, M. A. Popecki, K. N. Crocker, M. Granoff, Y. Jiang, E. Sartori,
V. Ye, H. Reme, J. A. Sauvaud, A. Cros, C. Aoustin, T. Camus, J.-L. Medale,
J. Rouzaud, C. W. Carlson, J. P. McFadden, D. Curtis, H. Heetderks, J.
Croyle, C. Ingraham, B. Klecker, D. Hovestadt, M. Ertl, F. Eberl, H. Kastle,
E. Kunneth, P. Laeverenz, E. Seidenschwang, E. G. Shelley, D. M. Klumpar,
E. Hertzberg, G. K. Parks, M. McCarthy, A. Korth, H. Rosenbauer, B. Grave,
L. Eliasson, S. Olsen, H. Balsiger, U. Schwab, M. Steinacher, The 3-D plasma
distribution function analyzers with time-of-flight mass discrimination
for Cluster, FAST, and Equator-S, AGU Monog. Meas. Techn. Space Plasmas,
ed. R. Pfaff, in press, 1998a.
Moebius, E. L. Tang,
L. M. Kistler, M. Popecki, E. J. Lund, D. Klumpar, W. Peterson, E. G. Shelley,
B. Klecker, D. Hovestadt, C. W. Carlson, R. Ergun, J. P. McFadden, F. Mozer,
M. Temerin, C. Cattell, R. Elphic, R. Strangeway, R. Pfaff, Species dependent
energies in upward directed ion beams over auroral arcs as observed with
FAST TEAMS, Geophys. Res. Lett., in press, 1998b.
Mozer, F. S., C.
W. Carlson, M. K. Hudson, R. B. Torbert, B. Parady, J. Yatteau, and M.
C. Kelley, Observations of paired electrostatic shocks in the polar magnetosphere,
Phys. Rev. Lett., 38, 292, 1977.
Persoon A. M., D.
A. Gurnett, W. K. Peterson, J. H. Waite, Jr., J. L. Burch, and J. L. Green,
Electron density depletions in the nightside auroral zone, J. Geophys.
Res., 93, 1871, 1988.
Strangeway, R. J.,
L. Kepko, R. C. Elphic, C. W. Carlson, R. E. Ergun, J. P. McFadden, W.
J. Peria, G. T. Delory, C. C. Chaston, M. Temerin, C. A. Cattell, E. Moebius,
L. M. Kistler, D. M. Klumpar, W. K. Peterson, E. G. Shelley, R. F. Pfaff,
FAST observations of VLF waves in the auroral zone: evidence of very low
plasma densities, Geophys. Res. Lett., submitted, 1998.
J. P. McFadden,
C. W. Carlson, R. E. Ergun, Space Sciences Laboratory, University of California,
Berkeley, CA 94720-7450. (e-mail: mcfadden@ssl.berkeley.edu)
D. M. Klumpar,
Lockheed Martin Palo Alto Research Laboratory, Palo Alto, CA 94304
E. Moebius,
L. Kistler, U. of New Hampshire, Durham, NH 93824
(Received May?,
1998; accepted?)
Copyright 1998 by the American Geophysical
Union.
Figure Captions
The figure shows
a comparison of the hot (>100 eV) electron and hot (>30 eV) ion densities
across an ion beam. Panels a and b show electron energy and pitch angle
spectrograms. Panels c and d show ion energy and pitch angle spectrograms.
Panels e, f and g are the integrated hot electron density, ion beam density
corrected for beam composition, and hot plasma sheet ion density. Panel
h is the ratio of ion to electron density, which is nearly one across the
ion beam.
Figure 2a is a
contour plot of the ion differential energy flux (proportional to count
rate) averaged over the beam in Figure 1. The 10 second average has broadened
the ion beam's parallel energy. Isotropic plasma sheet ions, minus the
loss cone, are seen at 10 keV. Counts below 30 eV are primarily due to
internally scattered inverted-V electrons, along with some scattered solar
ultraviolet (between 180o and 270o). These false
counts have a constant count rate below 30 eV (see Figure 2c) and produce
a spectra (Figure 2b) proportional to 1/energy. Figure 2d shows the partial
density (blue line,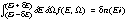 , where Ei is
the center energy of each energy step and
, where Ei is
the center energy of each energy step and 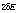 is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line,  , where
Emax is the highest energy step). From 2d it can be seen that the low energy
false counts would produce a ~20% error in the total density if included.
, where
Emax is the highest energy step). From 2d it can be seen that the low energy
false counts would produce a ~20% error in the total density if included.
Figure 3a is the
count rate from the FAST mass spectrometer (TEAMS) averaged over the same
10 second interval as Figure 2. No counts below 30 eV are measured by this
instrument, proving that the constant count rate seen at low energies in
Figure 2c is not real. The count rates for the nearly isotropic plasma
sheet ions (>5 keV) differ by a factor of two between Figures 3a and 2c,
as expected for the two instruments' relative geometric factors. The ion
beam is not prominent in the mass spectrometer data since it samples the
beam only twice per spin. The partial density calculation in Figure 3b
is nearly identical to Figure 2d except for the error at low energy (<30
eV) caused by false counts in the spectrometer data.
The figure shows
a comparison of hot (>100 eV) electron and hot (>30 eV) ion densities for
orbit 1676 with the same format as Figure 1. Panels e, f, and g show the
electron, ion beam and plasma sheet ion densities respectively. Panel h
shows the ratio of ion to electron density is near one during the ion beam.
Figure
5.
The figure shows
ion characteristics during the ion beam from Figure 4. The format is the
same as Figure 2 and displays many of the same characteristics. The ion
beam and plasma sheet ions are easily seen in Figure 5a. A weaker flux
of inverted-V electrons (see Figure 4) produces a smaller false count rate
in Figure 5c at energies <30 eV than is seen in Figure 2c.
Figure
6.
The figure shows
a comparison of hot (>100 eV) electron and hot (>30 eV) ion densities for
orbit 1771 with the same format as Figures 1 and 4. Panels e, f, and g
show the electron, ion beam and plasma sheet ion densities respectively.
Although the total density (panel e) varies by a factor of ~7 across this
beam event, the ratio of ion to electron density remains near one (panel
h).
Figure
7.
Contour plots of
the electron differential energy flux (proportional to count rate) both
inside (Figure 7a) and outside (Figure 7b) the ion beam region of Figure
1. Figures 7c and 7d show the partial density (blue line, 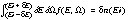 , where Ei is the center energy of each energy step and
, where Ei is the center energy of each energy step and 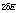 is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line, 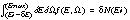 , where
Emax is the highest energy step) for the contour plots 7a and 7b, respectively.
Photoelectrons (<100 eV near -90o, 90o, 270o
pitch angles) dominate the density calculation if included in the sum.
Atmospheric secondary electrons are seen in Figure 7b at energies <200
eV and angles -50o to 50o and 130o to
230o, and secondary electrons produced in the analyzer are seen
at these angles at <50 eV in Figure 7a.
, where
Emax is the highest energy step) for the contour plots 7a and 7b, respectively.
Photoelectrons (<100 eV near -90o, 90o, 270o
pitch angles) dominate the density calculation if included in the sum.
Atmospheric secondary electrons are seen in Figure 7b at energies <200
eV and angles -50o to 50o and 130o to
230o, and secondary electrons produced in the analyzer are seen
at these angles at <50 eV in Figure 7a.
Orbit
# |
Ratio
Ni/Ne |
KP |
Electron
density
Range
(cm-3) |
Peak Energy
(kev) |
Ion
Beam
Density
(cm-3) |
O+/H+
Ratio* |
He+/H+
Ratio* |
Energy
range
(keV) |
PS
Density
(cm-3) |
PS
Temp
(keV) |
| 1525 |
1.13+/-0.11 |
1 |
.55-.85 |
1 |
.3-.6 |
0.28 |
0.67 |
.3-1. |
.35-.45 |
10 |
| 1616 |
0.99+/-0.08 |
1 |
1.2-2.5 |
1-2 |
.4-2.2 |
0.36 |
0.65 |
.1-1. |
.7-.8 |
5 |
| 1623 |
0.98+/-0.13 |
1- |
.9-2.5 |
.8 |
.45-2.3 |
0.54 |
0.34 |
.1-.3 |
.3 |
2 |
| 1633 |
1.00+/-0.14 |
1 |
.4-.7 |
2-3 |
.15-.6 |
1.28 |
0.47 |
.1-3 |
.15 |
5 |
| 1676 |
0.93+/-0.09 |
1- |
.33-.6 |
1.5 |
.15-.45 |
0.87 |
0.20 |
.1-2 |
.15-.2 |
3 |
| 1692 |
0.83+/-0.12 |
2+ |
.4-1.2 |
1-2 |
.15-.75 |
0.87 |
0.54 |
.3-2 |
.2-.35 |
6 |
| 1740 |
0.98+/-0.16 |
2- |
.13-.40 |
1-2 |
0.08-.2 |
1.09 |
0.38 |
1-2 |
0.04-.1 |
5 |
| 1761 |
0.98+/-0.16 |
1- |
.13-.28 |
2-3 |
.05-.1 |
1.44 |
0.46 |
1-5 |
0.06-.1 |
7 |
| 1771 |
0.88+/-0.13 |
1- |
.2-1.2 |
1-2 |
.15-.9 |
0.54 |
0.57 |
.3-2 |
.05-.15 |
3 |
| 1793** |
1.04+/-0.14 |
1 |
.25-1.0 |
3-10 |
.15-6. |
1.42 |
0.23 |
.3-20. |
.1-.25 |
10 |
| 1804 |
0.93+/-0.13 |
1 |
.2-1.0 |
1-10 |
.1-.5 |
1.11 |
0.31 |
.2-8 |
.05-.2 |
4 |
 /
/  ) since the ion spectrometer is not mass resolving. The plasma sheet ion
density is calculated assuming hydrogen, which is consistent with the mass
spectrometer data. The ion beam density has been calculated using a mass,
) since the ion spectrometer is not mass resolving. The plasma sheet ion
density is calculated assuming hydrogen, which is consistent with the mass
spectrometer data. The ion beam density has been calculated using a mass, 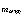 , given by:
, given by: /
/  ) since the ion spectrometer is not mass resolving. The plasma sheet ion
density is calculated assuming hydrogen, which is consistent with the mass
spectrometer data. The ion beam density has been calculated using a mass,
) since the ion spectrometer is not mass resolving. The plasma sheet ion
density is calculated assuming hydrogen, which is consistent with the mass
spectrometer data. The ion beam density has been calculated using a mass, 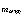 , given by:
, given by: . Panel h shows the ratio of hot ion to hot electron densities which shows
remarkably good agreement between the densities inside the ion beam even
though the beam density (panel f) varies by a factor of ~5 and the electron
density (panel f) varies by a factor of ~2.
. Panel h shows the ratio of hot ion to hot electron densities which shows
remarkably good agreement between the densities inside the ion beam even
though the beam density (panel f) varies by a factor of ~5 and the electron
density (panel f) varies by a factor of ~2.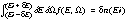 , where Ei
is the center energy of each energy step and
, where Ei
is the center energy of each energy step and 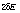 is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line, 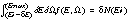 , where
Emax is the highest energy step). One can see that most of the density
resides in the hot component, however as much as 20% appears to exist in
the cold ions (4-30 eV). These low energy counts are about an order of
magnitude above the microchannel plate noise levels. However, as shown
below, these counts are due to a small number of scattered energetic electrons
and a small amount of solar ultraviolet and do not represent actual cold
plasma.
, where
Emax is the highest energy step). One can see that most of the density
resides in the hot component, however as much as 20% appears to exist in
the cold ions (4-30 eV). These low energy counts are about an order of
magnitude above the microchannel plate noise levels. However, as shown
below, these counts are due to a small number of scattered energetic electrons
and a small amount of solar ultraviolet and do not represent actual cold
plasma.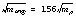 as determined
from the mass spectrometer data. The jump in plasma sheet ion density (panel
g) at 10:05:41-:45 and after 10:05:49 is caused by ion conics included
in the integral. Panel h shows that the ratio of hot ion to hot electron
density is about 1 when the ion beam is present.
as determined
from the mass spectrometer data. The jump in plasma sheet ion density (panel
g) at 10:05:41-:45 and after 10:05:49 is caused by ion conics included
in the integral. Panel h shows that the ratio of hot ion to hot electron
density is about 1 when the ion beam is present.
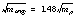 for the beam density calculation
(panel f). The plasma sheet ion density (panel g) is almost negligible
(~0.05 cm-3) for this event. Although the ion beam density (panel
f) varies by nearly a factor of 8, panel h shows that the ratio of hot
ion to hot electron densities remains near one across the event.
for the beam density calculation
(panel f). The plasma sheet ion density (panel g) is almost negligible
(~0.05 cm-3) for this event. Although the ion beam density (panel
f) varies by nearly a factor of 8, panel h shows that the ratio of hot
ion to hot electron densities remains near one across the event.
 .
.
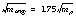 , varied by only +21% to -22% across the event due to additional changes
in He+/H+, and was relatively constant across most of the event giving
good agreement between ion and electron densities. A more sophisticated
program that uses a time varying,
, varied by only +21% to -22% across the event due to additional changes
in He+/H+, and was relatively constant across most of the event giving
good agreement between ion and electron densities. A more sophisticated
program that uses a time varying, 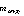 , across
the beams could have produced limited improvement in a couple of the density
ratio calculations but was unnecessary for this study. Table
1 provides theorists a range of parameters that can be used to model
the ion beam regions.
, across
the beams could have produced limited improvement in a couple of the density
ratio calculations but was unnecessary for this study. Table
1 provides theorists a range of parameters that can be used to model
the ion beam regions.
 in the density
calculation, a spectrometer determined density measurement that includes
low energy electrons requires close scrutiny. Figure 7c, formed from the
distribution in 7a within the ion beam, shows the partial electron density
at each energy step (blue line,
in the density
calculation, a spectrometer determined density measurement that includes
low energy electrons requires close scrutiny. Figure 7c, formed from the
distribution in 7a within the ion beam, shows the partial electron density
at each energy step (blue line,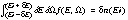 , where Ei
is the center energy of each energy step and
, where Ei
is the center energy of each energy step and  is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line, 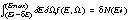 , where
Emax is the highest energy step). The contribution of photoelectrons and
internally scattered secondary electrons to the density clearly becomes
significant below 50 eV. The plateau of the green curve between 50 and
300 eV suggests minimal contribution of real electrons to the total density
at these low energies. This can be contrasted with Figure 7d, formed from
the distribution in 7b, which shows that atmospheric secondary electrons
between 50 and 300 eV contribute significantly to the total local density
outside ion beams.
, where
Emax is the highest energy step). The contribution of photoelectrons and
internally scattered secondary electrons to the density clearly becomes
significant below 50 eV. The plateau of the green curve between 50 and
300 eV suggests minimal contribution of real electrons to the total density
at these low energies. This can be contrasted with Figure 7d, formed from
the distribution in 7b, which shows that atmospheric secondary electrons
between 50 and 300 eV contribute significantly to the total local density
outside ion beams.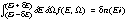 , where Ei is
the center energy of each energy step and
, where Ei is
the center energy of each energy step and 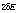 is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line,  , where
Emax is the highest energy step). From 2d it can be seen that the low energy
false counts would produce a ~20% error in the total density if included.
, where
Emax is the highest energy step). From 2d it can be seen that the low energy
false counts would produce a ~20% error in the total density if included.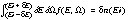 , where Ei is the center energy of each energy step and
, where Ei is the center energy of each energy step and 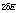 is the energy width), and the partial integral of the density starting
at the highest step (green line,
is the energy width), and the partial integral of the density starting
at the highest step (green line, 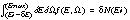 , where
Emax is the highest energy step) for the contour plots 7a and 7b, respectively.
Photoelectrons (<100 eV near -90o, 90o, 270o
pitch angles) dominate the density calculation if included in the sum.
Atmospheric secondary electrons are seen in Figure 7b at energies <200
eV and angles -50o to 50o and 130o to
230o, and secondary electrons produced in the analyzer are seen
at these angles at <50 eV in Figure 7a.
, where
Emax is the highest energy step) for the contour plots 7a and 7b, respectively.
Photoelectrons (<100 eV near -90o, 90o, 270o
pitch angles) dominate the density calculation if included in the sum.
Atmospheric secondary electrons are seen in Figure 7b at energies <200
eV and angles -50o to 50o and 130o to
230o, and secondary electrons produced in the analyzer are seen
at these angles at <50 eV in Figure 7a.