Figure 1: Total Al.1 emission for the 7-jan-1992 flare versus time.

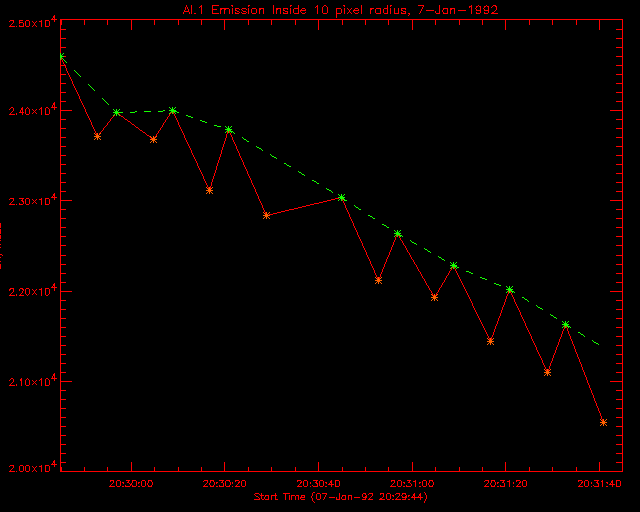
Figure 2 illustrates the ND filter effects. For this i'll use the 'radial profile', A(r), which is defined as the amount of emission inside a circular region, of radius r (in pixels) around the maximum. This plot is for the radial profile for region of r=10, for the last 18 images, which alternate between ND and OPEN filter configurations. The red line with the shows the actual values of A(10), while the dashed green line gives the values of A(10) interpolated using only the non-ND images. The positions of the ND images are shown by orange stars, and the non-ND images are shown by green stars. As you can see, there is less emission in the ND filter images. The average fractional shortfall for the ND images is: 0.027
Figure 2: The Emission within a 10 pixel radius of the maximum brightness versus time, for 7-jan-1992.
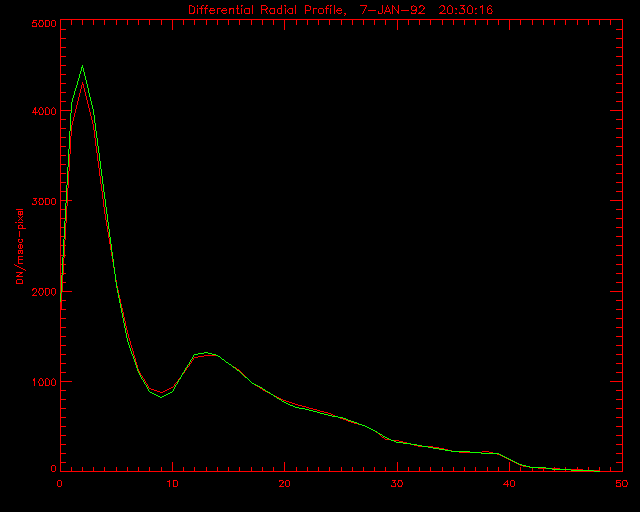
This next plot, Figure 3, shows dA(r) = A(r)-A(r-1), the differential radial profile, which gives a measure of the width of the image. The quantity dA(r) gives the amount of emission into a 1 pixel wide ring at radius r. This has not been normalized by the area of the ring. The red line shows the true profile for an ND image, and the green line is the value interpolated using the non-ND images. The profiles are similar, except that the ND filter value is lower by a couple of percent at the peak. The ND source is slightly wider too, by about 0.1 pixels.
The average fractional shortfall in Max da for the ND images is: 0.044 The average difference in the width of da for the ND images is: 0.110 pixels
Figure 3: The differential radial profile, 7-jan-1992, 20:30:16 UT.
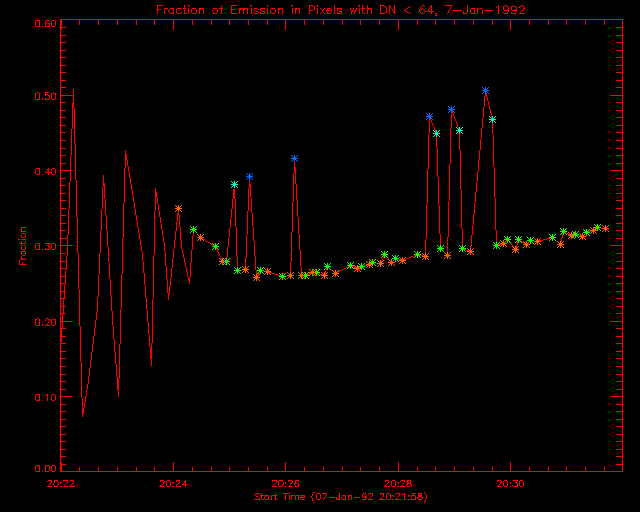
Here's another way to look at it. Figure 4 is a plot of the amount of brightness in each image that is concentrated in bright pixels; in this case in pixels with brightness greater than 103 DN/msec. Again, orange stars are the ND images, and green ones are the non-ND images, the green dashed line is interpolated using the non-ND images. The same thing happens; there is slightly less emission into the bright pixels for the ND images.
The average fractional shortfall for the ND images is: 0.061
Figure 4: Al.1 Emission for the brightest pixels versus time, 7-jan-1992.

PART 2) EXPOSURE TIME EFFECTS:
If you look back at the original plot (Fig. 1), you will note that the values of the total emission for the shortest exposures are less than the others. (These are the dark blue stars, with MBE=0, no ND filter, and an exposure time of 0.95 msec.). There are at least two effects that lead to this, and it's not clear which is dominant. The first is that for shorter exposures, there are more negative pixels included in the total emission. This next plot, Figure 5, is a time plot of the Background level: i.e., the average Dark Current Subtracted DN for the minimum 8x8 pixel region in each image. Note that we're making a distinction between 'Dark Current' and 'Background'. Dark Current is what's subtracted from the images by SXT_PREP. Background is what's left over, in the dimmest part of the image.
Figure 5: Background level, after dark current subtraction, 7-jan-1992.
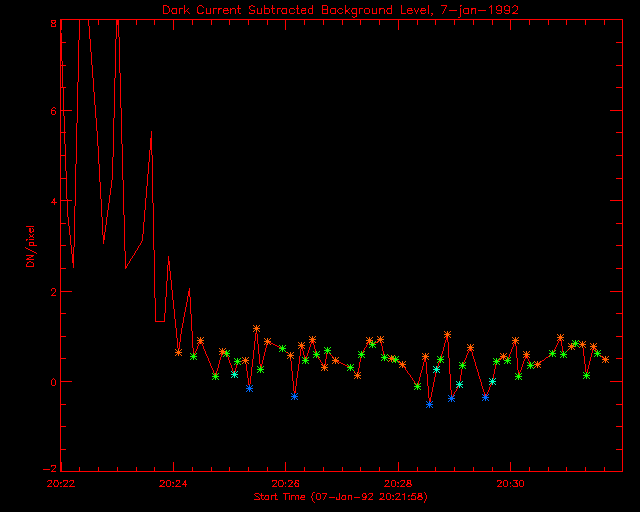
The different exposure levels are denoted by dark blue, light blue, green and orange stars as before. The shortest exposures have the lowest background level, and longer ones have higher levels. All of these images have had the same values of the dark current subtracted, a common happening before the entrance filter holes, so this is a reflection of the original data. This background is not due to scattered X-rays from the source, since it does not follow the flare time profile. (For large flares this 'background level' often does follow the flare profile for long exposures.)
The excess background could be X-rays from the active region behind the flare, or straylight. It is unlikely that this is an effect of time variation in the actual dark current; if dark current varied much with exposure time, we would expect it to vary with the exposure time before the correction for the ND filter. Instead this effect depends on the effective exposure time, after the ND correction. Thus the 1.4 msec, ND-filter images, which have non-corrected exposure times of 16 msec, (light blue stars), have smaller values then the 2.9 msec non-ND images (green stars).