The Cosmic-Ray Shadow and Coronal Magnetism
From RHESSI Wiki
| Nugget | |
|---|---|
| Number: | 351 |
| 1st Author: | Frederik Tenholt |
| 2nd Author: | |
| Published: | 13 May 2019 |
| Next Nugget: | Broken-up hard X-ray spectrum |
| Previous Nugget: | Kristian Birkeland |
| List all | |
Contents |
Introduction
Direct measurements of the coronal magnetic field are very difficult. We know something about the photospheric field, thanks mainly to the Zeeman effect, but normally one must rely on mathematical or MHD-based extrapolations to infer the structure of the coronal field. These models are physically incomplete and generally do not describe the current systems flowing in the corona and around the sources of the solar wind. Even the Parker Solar Probe, which has now successfully had two perilous perihelion passages, will reach its position closest to the Sun only in 2024.
This Nugget describes a relatively new diagnostic tool that provides information about this difficult region: the cosmic-ray shadow of the Sun. See also an earlier Nugget.
The cosmic-ray shadows of the Sun and Moon
Galactic and extragalactic cosmic rays are highly energetic charged particles reaching Earth from outer space. Their energy spectrum follows a power law over many orders of magnitude and the majority of cosmic rays are protons. Due to scattering in interestellar space, over the large distances of travel from their sources, the arrival directions of high-energy cosmic rays that reach Earth are to a great extent isotropic. At TeV (1012 eV) particle energies, many different air-shower experiments, but also other instruments such as the IceCube Neutrino Observatory have measured the cosmic-ray shadows of the Moon and the Sun quite well.
While the Moon is an inert mass, best used for calibration purposes, the cosmic-ray shadow of the Sun can be used in order to learn about the highly variable solar magnetic field. The coronal magnetic field bends the paths of the cosmic rays in a predictable manner due to the Lorentz force. In simple terms, the ingredients for the cosmic-ray shadow of the Sun are the cosmic rays themselves, the Sun and its magnetic field, and the detection of cosmic rays at Earth. Assuming that the cosmic rays and their detection on Earth are more or less well-known, one can therefore draw conclusions about the Suns magnetic field from observing the shadow.
Simulations
In our recent work (Ref. [0]), we computed the cosmic-ray shadow of the Sun in the energy range of 5 to 316 TeV for five different particle masses and for different sets of Carrington rotations. For the coronal magnetic field, we assume a field derived from the Potential Field Source Surface (PFSS) model. Figure 1 shows an example of one of our modeled cosmic-ray images, revealing the distortions produced by the assumed coronal magnetic field.
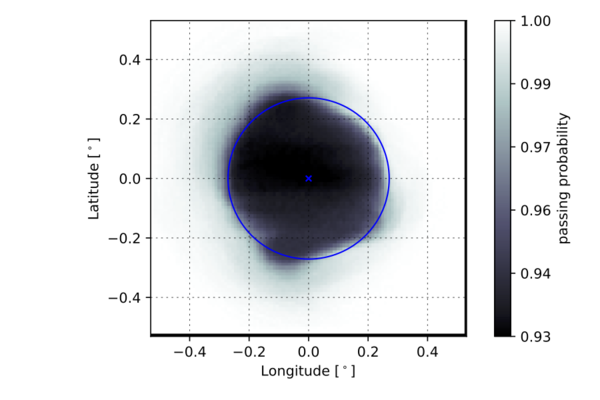
We also tested our simulations in an artificial magnetic field representing an ideal dipole. In order to reduce computational time, we only propagate cosmic rays that will in the end reach the Earth. This is achieved by a so-called back-tracking algorithm, which uses the principle that anti-particles behave the same as particles when propagated backwards in time.
Our calculations predict a time-variation of the solar shadow and hint at an anti-correlation with solar activity. The stronger and more irregular the solar magnetic field, the weaker the shadow, and vice versa. This is in agreement with recent experimental results (Ref. [3]) and is an interesting independent measure for the structure of the solar magnetic field.
Additionally, in the extreme case of an ideal dipole field or for times of very low solar activity, we predict a peak structure of the depth of the Sun shadow as a function of particle rigidity. This rigidity- (or energy-) dependent behavior of the Sun shadow (Figure 2) has not been observed yet

Summary
The cosmic-ray shadow of the Sun as measured by several experiments is a relatively new, but powerful diagnostic tool for evaluating different models of the coronal magnetic field close to the Sun. An energy-dependent measurement of the shadow even further enhances the possibilities for quantitative studies.
References
[1] "Arrival Directions of Cosmic-Ray Air Showers from the Northern Sky"
[3] "Detection of the Temporal Variation of the Sunʼs Cosmic Ray Shadow with the IceCube Detector"
| RHESSI Nugget Date | 13 May 2019 + |
| RHESSI Nugget First Author | Frederik Tenholt + |
| RHESSI Nugget Index | 351 + |